The figure at right is a pentagram. A pentagram is a 5‑pointed star that has congruent angles at each of its outer vertices.

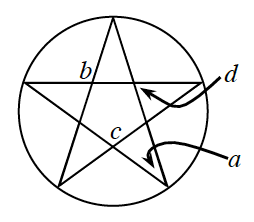
1. Use the fact that all pentagrams can be inscribed in a circle to find the measure of angle $a$ at right.

   Step 1(a):

   Find the measure of each of the intercepted arcs.

   $\frac{360^\circ}{5} = 72^\circ$

   Step 2(a):

   The degree measure of an inscribed angle is half the measure of the intercepted arc.

   $\frac{72^\circ}{2}$

   Answer (a):

   $=36^\circ$

2. Find the measure of angles $b$, $c$, and $d$.

   Hint (b):

   Use the information found in part (a) and remember that a pentagram has a regular pentagon inside of it, and the angles in a regular pentagon are equal.